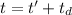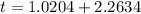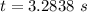Answer:
a)
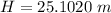
b)
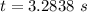
Step-by-step explanation:
Given:
- mass of the ball thrown up,
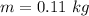
- initial velocity of the ball thrown up,
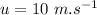
- height above the ground from where the ball is thrown up,
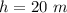
a)
Maximum height attained by the ball above the roof level can be given by the equation of motion.
As,

where:
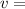 final velocity at the top height of the upward motion
final velocity at the top height of the upward motion
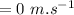
 acceleration due to gravity
acceleration due to gravity
 height of the ball above the roof
height of the ball above the roof
Now,
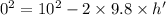
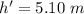
Therefore total height above the ground:

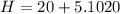
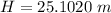
b)
Now we find the time taken in raching the height
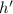 :
:
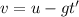
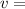 final velocity at the top of the motion
final velocity at the top of the motion
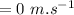
So,
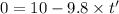
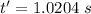
Now the time taken in coming down to the ground from the top height:

where:
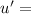 is the initial velocity of the ball in course of coming down to ground from the top
is the initial velocity of the ball in course of coming down to ground from the top
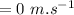
Here the direction acceleration due to gravity is same as that of motion so we are taking them positively.
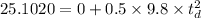
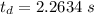
Therefore the total time taken in by the ball to hit the ground after it begins its motion: