Question

The rectangle shown has a perimeter of 56 cm and the given area it's length is 7 more than twice it's with right inside of a system of equations to find that dimensions

Answer 1

The dimensions are length 7 cm and width 21 cm.

Explanation:

Given:

The rectangle shown has a perimeter of 56 cm.

It's length is 7 more than twice its width.

Now, to find the dimensions.

Let the width be
`x.`

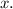
And the length =
`7+2x.`

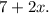
Perimeter = 56 cm.

Now, putting formula to get the dimensions:

`Perimeter=2(length+width)`

`56=2((7+2x)+x)`

`56=2(7+2x+x)`

`56=2(7+3x)`

`56=14+6x`

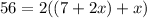
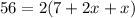
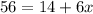
Subtracting both sides by 14 we get:

`42=6x`

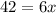
Dividing both sides by 6 we get:

`7=x\\x=7\ cm.`

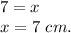
So, the width = 7 cm.

And the length:

7+2
`x`

= 7 + 2\times 7

= 7 + 14

= 21 cm.

Therefore, the dimensions are length 7 cm and width 21 cm.