Question

Match the integrals with the type of coordinates which make them the easiest to do. Put the letter of the coordinate system to the left of the number of the integral.

1. \displaystyle \int_{0}^{1} \! \int^{y^2}_{0} \ \frac{1}{x} \ dx \ dy
2. \displaystyle \int \!\! \int \!\! \int_E \ z \ dV where E is: 1 \leq x \leq 2, \ \ 3 \leq y \leq 4, \ \ 5 \leq z \leq 6
3. \displaystyle \int \!\! \int \!\! \int_E \ z^2 \ dV where E is: -2 \leq z \leq 2, \ \ 1 \leq x^2+y^2 \leq 2
4. \displaystyle \int \!\! \int \!\! \int_E \ dV where E is: x^2+y^2+z^2 \leq 4, \ \ x \geq 0, \ \ y \geq 0, \ \ z \geq 0
5. \displaystyle \int \!\! \int_D \ \frac{1}{x^2+y^2} \ dA where D is: x^2+y^2 \leq 4


A. polar coordinates
B. spherical coordinates
C. cylindrical coordinates
D. cartesian coordinates

Answer 1

D 1

D 2

C 3

B 4

A 5

Explanation:

for

`\displaystyle \int_(0)^(1) \! \int^(y^2)_(0) \ (1)/(x) \ dx \ dy`

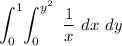
then Cartesian coordinates are easier to use since 1/x and y² are easy to integrate.

for

`\displaystyle \int \!\! \int \!\! \int_E \ z \ dV where E is: 1 \leq x \leq 2, \ \ 3 \leq y \leq 4, \ \ 5 \leq z \leq 6`

then Cartesian coordinates are easier to use since each integral is not dependent on the others

for

`\displaystyle \int \!\! \int \!\! \int_E \ z^2 \ dV where E is: -2 \leq z \leq 2, \ \ 1 \leq x^2+y^2 \leq 2`

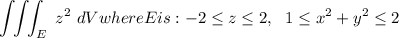
then cylindrical coordinates are easier to use since

x²+y²=r² → 1≤r≤√2 , 0≤θ≤2*π

-2≤z≤2

therefore each integral is not dependent on the others and with the transformation the functions do not get more complex to integrate → is easier to solve

for

`\displaystyle \int \!\! \int \!\! \int_E \ dV where E is: x^2+y^2+z^2 \leq 4, \ \ x \geq 0, \ \ y \geq 0, \ \ z \geq 0`

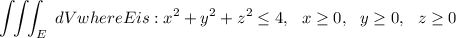
then spherical coordinates are easier to use since

x²+y²+z²=ρ² → ρ≤√2 ,

x≥0, y≥0,z≥0 → 0≤θ≤π , 0≤φ≤π/2

therefore each integral is not dependent on the others and with the transformation the functions do not get more complex to integrate → is easier to solve

for

`\displaystyle \int \!\! \int_D \ (1)/(x^2+y^2) \ dA where D is: x^2+y^2 \leq 4`

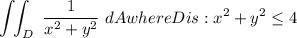
then polar coordinates are easier to use since

x²+y²=r² → r≤2 , 0≤θ≤2*π

therefore each integral is not dependent on the others and with the transformation the functions get even easier to integrate (1/x²+y² turns to 1/r² that is easily integrable) → is easier to solve