Answer:
range of the given function in Interval notation is [-1, 3]
Explanation:
i) given function is y = 1 + 2sin(x - 11)
ii) maximum of sin function is 1
iii) therefore maximum of given function
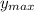 = 1 + (2
= 1 + (2
 1) = 3
1) = 3
iv) minimum of sin function is -1
v) therefore maximum of given function
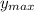 = 1 + (2
= 1 + (2
 -1) = -1
-1) = -1
vi) therefore the range of the given function in Interval notation is [-1,3]