Question

(havemercy)

A basketball player shoots a basketball with an initial velocity of 15 ft/sec. The ball is released from an initial height of 6.5 feet. The function
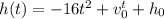
`h(t) = -16t^(2) + v_(0)^(t) + h_(0)` models the height, in feet, of an object after t seconds.
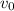
`v_(0)` is the initial velocity of the object, and
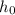
`h_(0)` is the initial height of the object.
Part 1: Write a function that models the height of the basketball. Use your function to answer Parts 2-4.
Part 2: How long does it take for the basketball to hit the ground? Round your answer to the nearest hundredth. Show all of your work. You're welcome to use this quadratic formula calculator, but please explain your answer. (Links to an external site.)
Part 3: When does the basketball reach its maximum height? Round your answer to the nearest hundredth. Show all of your work and explain your answer.
Part 4: What is the maximum height of the basketball? Round your answer to the nearest hundredth. Show all of your work and explain your answer.


A:

B:

C:

D:

Answer 1

Part 1: the function would be

h(t)= -16t^2+15t+6.5

Part 2: using the same steps it stated on the calculator

x=1.26

Part 3: by using t= -b/(2a) 15 as b and -16 as a makes it

t= 15/32

Part 4: You then put 15/32 in for t in the equation making the maximum height

10.02

IDK IF THIS IS RIGHT BUT IVE SPENT ALL DAY ON THIS