Question

A bouquet of lilies and tulips has 20 flowers. Lilies cost 3$ each and tulip cost 2$ each. The bouquet costs $48 how many lilies x and tulips y are in a bouquet? Show how you got your equations and answers.

Answer 1

8 lilies and 12 tulips are in bouquet

Solution:

Let "x" be the number of lilies

Let "y" be the number of tulips

Cost of 1 lily = $ 3

Cost of 1 tulip = $ 2

A bouquet of lilies and tulips has 20 flowers

Therefore,

number of lilies + number of tulips = 20

x + y = 20

y = 20 - x ---------- eqn 1

The bouquet costs $48. Therefore, we frame a equation as:

number of lilies x Cost of 1 lily + number of tulips x number of tulips = 48

`x * 3 + y * 2 = 48`

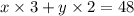
3x + 2y = 48 -------- eqn 2

Let us solve eqn 1 and eqn 2

Substitute eqn 1 in eqn 2

3x + 2(20 - x) = 48

3x + 40 - 2x = 48

x = 8

Substitute x = 8 in eqn 1

y = 20 - 8

y = 12

Thus 8 lilies and 12 tulips are in bouquet