Answer:
4) 27, 32, 45 is NOT a Pythagorean triplet.
Explanation:
Here the given triangle is a Right Triangle.
Now,a s we know, if a , b are the two sides, and c is the hypotenuse, then:
by PYTHAGORAS THEOREM:
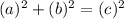
Also, Hypotenuse is ALWAYS the longest side in a right triangle.
Consider the given cases, and check for Pythagoras theorem:
1) 12, 16, 20
Here, a = 12, b = 16 and c = 20
a² + b² = (12)² + (16)² = 144+ 256 = 400 = (20)² = (c)²
⇒ a² + b² = (c)²
Hence, 12, 16, 20 is a Pythagorean triplet.
2) 10, 24, 26
Here, a = 10, b = 24 and c = 26
a² + b² = (10)² + (24)² = 100+ 576 = 676 = (26)² = (c)²
⇒ a² + b² = (c)²
Hence, 10, 24, 26 is a Pythagorean triplet.
3) 14, 48, 50
Here, a = 14, b = 48 and c = 50
a² + b² = (14)² + (48)² = 196+ 2304 = 2500 = (50)² = (c)²
⇒ a² + b² = (c)²
Hence, 14, 48, 50 is a Pythagorean triplet.
4) 27, 32, 45
Here, a = 27, b = 32 and c = 45
a² + b² = (27)² + (32)² = 729+ 1024 = 1753 ≠ (45)² = 2025 = (c)²
⇒ a² + b² ≠ (c)²
Hence, 27, 32, 45 is NOT a Pythagorean triplet.