Question

Justin likes to skate at an ice cream parlor that is due south of his school and due west of his favorite candy store. If the ice cream parlor is 2 miles from his school and the straight-line distance between the school and the candy is 5 miles, how far is the ice cream parlor from the candy store? If necessary, round to the nearest tenth.

Answer 1

Distance between ice-cream parlor and candy store is 4.6 miles.

Explanation:

We have drawn the diagram for your reference.

Given:

ice cream parlor that is due south of his school at 2 miles.

So According to diagram:

SI = 2 miles

Also Given:

school and the straight-line distance between the school and the candy is 5 miles which is in west.

SC = 5 miles

We need to find the distance between ice-cream parlor and candy store.

According to diagram We need to find IC.

Solution:

Let us assume Δ ISC to be right angled triangle.

Then we will apply Pythagoras theorem we get;

`SC^2=IC^2+SI^2\\\\IC^2=SC^2-SI^2`

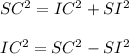
Substituting the values we get;

`IC^2 = 5^2-2^2\\\\IC^2=25-4 =21`

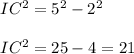
Now taking square root on both side we get;

`√(IC^2) =√(21) \\\\IC = 4.582\ miles`

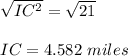
Rounding to nearest tenth we get;

`IC=4.6\ miles`

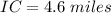
Hence distance between ice-cream parlor and candy store is 4.6 miles.