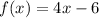Answer
The equation of line in function notation is:
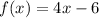
Step-by-step explanation:
Given points:
(3,6) and (4,10)
To find the equation of the line in function notation.
Solution:
In order to find the equation of the line we will first find the slope of the line.
The slope of a line passing through points
 and
and
 the slope can be given as:
the slope can be given as:
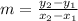
Plugging in the given points to find the slope of the line.
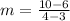
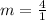
∴
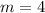
Equation of line can be written in point slope form as:

where
 is a point on the line.
is a point on the line.
Using point (3,6)

Using distribution:
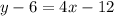
Adding 6 both sides.
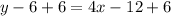
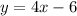 [Equation of line]
[Equation of line]
To write the equation in function notation, we will replace
 with
with
 .
.
So, the equation of the line in function notation can be given as: