The area of the metal frame is
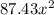 sq. units.
sq. units.
Solution:
A circular mirror is surrounded by a square metal frame.
Radius of the circular mirror = 2x
Side length of the square frame = 10x
To find the area of the metal frame.
Area of the square frame = Side × Side
=
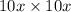
=
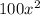 sq. units
sq. units
Area of the square metal frame =
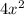 sq. units
sq. units
Area of the circular mirror =

=
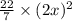
=
 sq. units
sq. units
Area of the circular mirror =
 sq. units
sq. units
Area of the metal frame = Area of the square frame – Area of the mirror
=

=
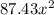 sq. units
sq. units
Hence the area of the metal frame is
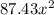 sq. units.
sq. units.