The solution to given inequality is: x < -1
Solution:
Given inequality is:
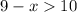
We have to solve the inequality for "x"
Let us solve the inequality step by step

The important rule in inequality is:
Whenever you multiply or divide an inequality by a negative number, you must flip the inequality sign.
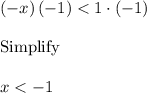
Thus the solution to given inequality is:
