The missing numbers: y = –23x + 22
Solution:
Let us find the linear equation to the given data.
To find y in terms of x.
First calculate the common difference for y.
–47 – (–24) = –23
–70 – (–47) = –23
–93 – (–70) = –23
So, the common difference = –23
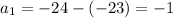
General expression for a linear equation using arithmetic sequence.
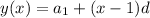
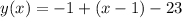
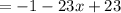
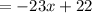

Hence the missing numbers: y = –23x + 22.