Answer:
Explanation:
Given : In a state where license plates contain six digits.
Probability of that a number is 9 =
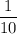 [Since total digits = 10]
[Since total digits = 10]
We assume that each digit of the license number is randomly selected .
Since each digit in the license plate is independent from the other and there is only two possible outcomes for given case (either 9 or not), so we can use Binomial.
Binomial probability formula:
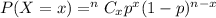
, where n= total trials , p = probability for each success.
Let x be the number of 9s in the license plate number.

Then, the probability that the license number of a randomly selected car has exactly two 9's will be :

Hence, the required probability = 0.098415