Question

An SRS of 350 high school seniors gained an average of x¯=21 points in their second attempt at the SAT Mathematics exam. Assume that the change in score has a Normal distribution with standard deviation σ=52 .(a) Find a 99% confidence interval for the mean change in score μ in the population of all high school seniors. (Enter your answers rounded to two decimal places.)lower bound of confidence interval: ???upper bound of confidence interval: ???(b) What is the margin of error for 99% ? (Enter your answer rounded to two decimal places.)margin of error: ????(c) Suppose we had an SRS of just 100 high school seniors. What would be the margin of error for 99% confidence? (Enter your answer rounded to three decimal places.)margin of error: ????(d) How does decreasing the sample size change the margin of error of a confidence interval when the confidence level and population standard deviation remain the same?A. Decreasing the sample size keeps the margin of error the same, provided the confidence level and population standard deviation remain the same.B. Decreasing the sample size increases the margin of error, provided the confidence level and population standard deviation alsodecrease.C. Decreasing the sample size decreases the margin of error, provided the confidence level and population standard deviation remain the same.D. Decreasing the sample size increases the margin of error, provided the confidence level and population standard deviation remain the same.

Answer 1

To find a 99% confidence interval for the mean change in score in the population of all high school seniors, we can use the formula: Confidence Interval = sample mean ± (critical value * (population standard deviation / square root of sample size)). The margin of error is the critical value times the standard deviation divided by the square root of the sample size. Decreasing the sample size while keeping the confidence level and population standard deviation constant increases the margin of error.

Step-by-step explanation:

To find a 99% confidence interval for the mean change in score in the population of all high school seniors, we can use the following formula:

Confidence Interval = sample mean ± (critical value * (population standard deviation / square root of sample size))

First, calculate the critical value for a 99% confidence level, which corresponds to a z-score of approximately 2.576. Then substitute the values into the formula to find the confidence interval. The lower bound of the confidence interval is the sample mean minus the margin of error, and the upper bound is the sample mean plus the margin of error.

(a) lower bound of confidence interval: 21 - (2.576 * (52 / square root of 350))
upper bound of confidence interval: 21+ (2.576 * (52 / square root of 350))

(b) The margin of error for 99% confidence is the critical value times the standard deviation divided by the square root of the sample size: 2.576 * (52 / square root of 350)

(c) To find the margin of error for 99% confidence with a sample size of 100, substitute the values into the formula: 2.576 * (52 / square root of 100)

(d) Decreasing the sample size while keeping the confidence level and population standard deviation constant increases the margin of error. This means that the range of the confidence interval becomes wider, providing less precision in estimating the true population mean.

Answer 2

a)
`21-2.58(52)/(√(350))=13.83`

`21+2.58(52)/(√(350))=28.17`

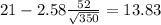
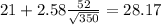
So on this case the 99% confidence interval would be given by (13.83;28.17)

b)
`ME=2.58(52)/(√(350))=7.17`

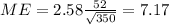
c)
`ME=2.58(52)/(√(100))=13.42`

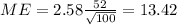
d) D. Decreasing the sample size increases the margin of error, provided the confidence level and population standard deviation remain the same.

Step-by-step explanation:

Previous concepts

A confidence interval is "a range of values that’s likely to include a population value with a certain degree of confidence. It is often expressed a % whereby a population means lies between an upper and lower interval".

The margin of error is the range of values below and above the sample statistic in a confidence interval.

Normal distribution, is a "probability distribution that is symmetric about the mean, showing that data near the mean are more frequent in occurrence than data far from the mean".

Part a

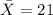`\bar X=21` represent the sample mean

`\mu` population mean (variable of interest)

`\sigma=52` represent the population standard deviation

n=350 represent the sample size

99% confidence interval

The confidence interval for the mean is given by the following formula:

`\bar X \pm z_(\alpha/2)(\sigma)/(√(n))` (1)

Since the Confidence is 0.99 or 99%, the value of
`\alpha=0.01` and
`\alpha/2 =0.005`, and we can use excel, a calculator or a table to find the critical value. The excel command would be: "=-NORM.INV(0.005,0,1)".And we see that
`z_(\alpha/2)=2.58`

Now we have everything in order to replace into formula (1):

`21-2.58(52)/(√(350))=13.83`

`21+2.58(52)/(√(350))=28.17`

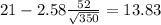
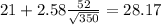
So on this case the 99% confidence interval would be given by (13.83;28.17)

Part b

The margin of error is given by:

`ME=2.58(52)/(√(350))=7.17`

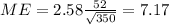
Part c

The margin of error is given by:

`ME=2.58(52)/(√(100))=13.42`

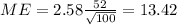
Part d

As we can see when we reduce the sample size we increase the margin of error so the best option for this case is:

D. Decreasing the sample size increases the margin of error, provided the confidence level and population standard deviation remain the same.