Question

6.3.AP-9

3 Question Help
Suppose that you have $6,000 to invest. Which investment yields the greater return over a 10 year period: 7.42% compounded daily or 7.5% compounded quarterly?
Find the total amount of the investment after 10 years if $6,000 is invested at 7.42% compounded daily.
$
(Round to the nearest cent as needed.)​

Answer 1

The investment at 7.42%; $12 599.84

Explanation:

The formula for the amount (A) accrued on an investment earning compound interest is

`A = P(1 + (r)/(n))^(nt)`

where

P = the amount of money invested (the principal)

r = the annual interest rate expressed as a decimal fraction

t = the time in years

n = the number of compounding periods per year

1. Daily compounding

Data:

P = $6000

r = 7.42 % = 0.0742

t = 10 yr

n = 365 /yr

Calculation:

`\begin{array}{rcl}\\A& =& P \left (1 + (r)/(n) \right )^(nt)\\\\& =& 6000 \left(1 + (0.0742)/(365) \right)^(365*10)\\\\& =& 6000 \left(1 + 0.0002032888 \right)^(365*10)\\& =& 6000 (1.000203288)^(3650)\\& =& 6000 * 2.0999732\\& =& \mathbf{12599.84}\\\end{array}\\`

2. Quarterly compounding

Data:

r = 7.5 % = 0.075

n = 4 /yr

Calculation:

`\begin{array}{rcl}A& =& P \left (1 + (r)/(n) \right )^(nt)\\& =& 6000 \left(1 + (0.075)/(4) \right )^(4*10)\\& =& 6000 (1 + 0.01875)^(40)\\& =& 6000 (1.01875)^(40)\\& =& 6000 * 2.102349277\\& =& \mathbf{12614.10}\\\end{array}\\`

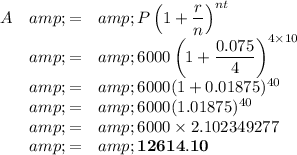
This investment yields the greater return, but the difference is less than $1.50 per year.

3. $ 6000 at 7.42 % compounded daily

From Part 1, the value of the investment is $12 599.84.