Question

The graph of f(x) = One-half(2.5)x and its reflection across the x-axis, g(x), are shown. On a coordinate plane, 2 exponential functions are shown. f (x) approaches y = 0 in quadrant 2 and then increases into quadrant 1. It crosses the y-axis at (0, 0.5) and goes through (2, 3). g (x) approaches y = 0 in quadrant 3 and decreases into quadrant 4. It crosses the y-axis at (0, negative 0.5) and goes through (2, negative 3). What is the range of g(x)? all real numbers all real numbers less than 0 all real numbers greater than 0 all real numbers less than or equal to 0

Answer 1

The awnser is B

Explanation:

So you dont have to read all that above

Answer 2

The range of g(x) will be all real numbers less than zero.

Explanation:

The graph of
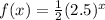`f(x) = (1)/(2)(2.5)^(x)` and its reflection across the x-axis, g(x), are given.

Now, another function g(x) will be given by
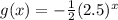`g(x) = - (1)/(2)(2.5)^(x)` ............ (1)

Since by reflection across the x-axis the curve will change its y-value only by sign and the x-value remains the same.

Now, g(x) approaches y = 0 in quadrant 3 and decreases into quadrant 4. It crosses the y-axis at (0,-0.5) and goes through (2,-3).

Therefore, the range of the equation (1) will be all real numbers less than zero. (Answer)