Question

In square ABCD, point M is the midpoint of side AB and point N is the midpoint of side BC. What is the ratio of the area of triangle AMN to the area of square ABCD? Express your answer as a common fraction.

Answer 1

1:8

Explanation:

Given that in square ABCD, point M is the midpoint of side AB and point N is the midpoint of side BC.

Let the side of the square be a.

Area of square ABCD =
`a^2`

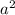
The triangle AMN is having two legs of a right triangle as half of side of the square

i.e. Triangle AMN has base = height = a/2

So area of triangle AMN =
`(1)/(2) bh\\=(a^2)/(8)`

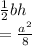
Ratio of the area of triangle AMN to area of square ABCD

= 1:8