Question

Two masses, A and B, are attached to different springs. Mass A vibrates with an amplitude of 8 cm at a frequency of 10 Hz and mass B vibrates with an amplitude of 5 cm at a frequency of 16 Hz. How does the maximum speed of A compare to the maximum speed of B

Answer 1

To solve this problem we will apply the concepts of speed given the simple harmonic movement, for which it defines this speed as

`v_(max) = \omega A`

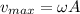
Here

`\omega =`Angular velocity

A = Amplitude

Recall that the angular velocity is equivalent in terms of the frequency at

`\omega = 2\pi f`

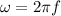
If we replace the value we will have then

`v_(max) = 2\pi f A`

For mass A

`v_(max,A) = 2\pi (10)(0.08) = 5.024m/s`

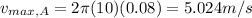
For mass B

`v_(max.B) = 2\pi (16)(0.05) = 5.024m/s`

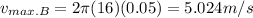
Therefore they are equal.