Question

Historically, the proportion of people who trade in their old car to a car dealer when purchasing a new car is 48%. Over the previous 6 months, in a sample of 115 new-car buyers, 46 have traded in their old car. To determine (at the 10% level of significance) whether the proportion of new-car buyers that trade in their old car has statistically significantly decreased; what is the critical value

Answer 1

Answer: The proportion of new car buyers that trade in their old car has statistically significantly decreased.

Explanation:

Since we have given that

p = 48% = 0.48

n = 115

x = 46

So,
`\hat{p}=(46)/(115)=0.40`

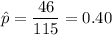
So, hypothesis would be

`H_0:\ p=\hat{p}\\\\H_a:p<\hat{p}`

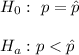
So, test value would be

`z=\frac{p-\hat{p}}{\sqrt{(p(1-p))/(n)}}\\\z=\frac{0.48-0.40}{\sqrt{(0.48* 0.52)/(115)}}\\\\z=(0.08)/(0.0466)\\\\z=1.72`

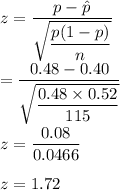
At 10% level of significance, critical value would be

z= 1.28

Since 1.28 < 1.72

So, we will reject the null hypothesis.

Hence, the proportion of new car buyers that trade in their old car has statistically significantly decreased.