Question

A balloon filled with 0.25 mol of He(g) at 273 K and 1 atm is allowed to rise through the atmosphere. Which of the following explains what happens to the volume of the balloon as it rises from ground level to an altitude where the air temperature is 220 K and the air pressure is 0.1 atma. The volume will increase because the decrease in air pressure will have a greater effect than the decrease in temperature. b. The volume will remain unchanged because of the counteracting effects of the decrease in temperature and the decrease in air pressure c. The volume will decrease because the decrease in temperature will have a greater effect than the decrease in air pressure d. It cannot be determined whether the volume of the balloon will increase, decrease, or remain the same without knowing the initial volume of the balloon.

Answer 1

#13 is your correct answer on attached photo

Step-by-step explanation:

Answer 2

a. The volume will increase because the decrease in air pressure will have a greater effect than the decrease in temperature.

Step-by-step explanation:

Hello,

In this case, we could predict the resulting volume numerically by using the combined ideal gas relationship as shown below:

`(p_1V_1)/(T_1)=(p_2V_2)/(T_2)`

Now, the volume as the first state is computed form the ideal gas law:

`V_1=(n_1RT_1)/(p_1)=(0.25mol*0.082(atm*L)/(mol*K)*273K)/(1atm) =5.5965L`

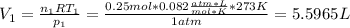
Thus, for the given conditions, we must solve for the volume at the second state:

`V_2=(p_1V_1T_2)/(T_1p_2)=(1atm*5.5965L*220K)/(0.1atm*273K)\\V_2=45.1L`

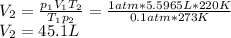
Such volume, clearly is explained by: a. The volume will increase because the decrease in air pressure will have a greater effect than the decrease in temperature.

Best regards.