Answer:
H1:
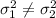
So then the correct option for this case would be:
H1:σ21≠σ22
Explanation:
Data given and notation
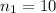 represent the sampe size for professor 1
represent the sampe size for professor 1
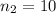 represent the sample size for professor 2
represent the sample size for professor 2
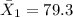 represent the sample mean for professor 1
represent the sample mean for professor 1
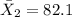 represent the sample mean for professor 2
represent the sample mean for professor 2
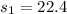 represent the sample deviation for professor 1
represent the sample deviation for professor 1
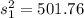 represent the sample variance for professor 1
represent the sample variance for professor 1
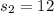 represent the sample deviation for professor 2
represent the sample deviation for professor 2
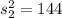 represent the sample variance for professor 2
represent the sample variance for professor 2
 represent the significance level provided
represent the significance level provided
F test is a statistical test that uses a F Statistic to compare two population variances, with the sample deviations s1 and s2. The F statistic is always positive number since the variance it's always higher than 0. The statistic is given by:
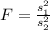
Solution to the problem
System of hypothesis
We want to test if the variation between their grading procedure, so the system of hypothesis are:
H0:
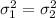
H1:
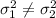
So then the correct option for this case would be:
H1:σ21≠σ22
Calculate the statistic
Now we can calculate the statistic like this:
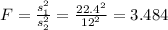
Now we can calculate the p value but first we need to calculate the degrees of freedom for the statistic. For the numerator we have
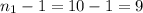 and for the denominator we have
and for the denominator we have
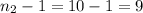 and the F statistic have 9 degrees of freedom for the numerator and 9 for the denominator. And the P value is given by:
and the F statistic have 9 degrees of freedom for the numerator and 9 for the denominator. And the P value is given by:
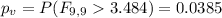
And we can use the following excel code to find the p value:"=1-F.DIST(3.484,9,9;TRUE)"
Conclusion
Assuming
 and since the
and since the
 we have enough evidence to reject the null hypothesis.
we have enough evidence to reject the null hypothesis.