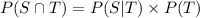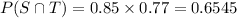Question

A car repair is either on time or late and either satisfactory or unsatisfactory. If a repair is made on time, then there is a probability of 0.85 that it is satisfactory. There is a probability of 0.77 that a repair will be made on time. What is the probability that a repair is made on time and is satisfactory?

Answer 1

0.6545

Explanation:

Let
`P(T)` be probability of being on time and
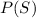`P(S)` the probability of being satisfactory. We have conditional probability here since being satisfactory depends on being on time. Conditional probability states

`P(S|T) =(P(S\cap T))/(P(T))`

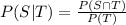
where

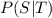`P(S|T)` is the probability of
`S` given that
`T` has occurred;

`P(S\cap T)` is the probability of
`S` and
`T`.

Rearranging, we have

`P(S\cap T)=P(S|T)* P(T)`

`P(S\cap T)=0.85*0.77=0.6545`