Question

Pulse rates of women are normally distributed with a mean of 77.5 beats per minute and a standard deviation of 11.6 beats per minute. Answer the following questions.What are the values of the mean and standard deviation after converting all pulse rates of women to z scores usingz = (x-mean)/ standard dev.

Answer 1

Mean = 0, Standard deviation = 1

Explanation:

We are given the following in the question:

Mean, μ = 77.5 beats per minute

Standard Deviation, σ = 11.6 beats per minute

We are given that the distribution of pulse rates of women is a bell shaped distribution that is a normal distribution.

Formula:

`z_(score) = \displaystyle(x-\mu)/(\sigma)`

Putting the values, we get,

`z_(score) = \displaystyle(x-77.5)/(11.6)`

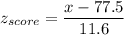
After, the standardization, that is conversion of all pulse rates of women to z scores, the mean of the distribution is 0 and standard deviation is 1.

Mean = 0, Standard deviation = 1