Answer: 0.205 m/s
Step-by-step explanation:
According the conservation of momentum law, the total momentum before the collision (
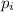 ) is equal to the total momentum after the collision (
) is equal to the total momentum after the collision (
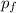 ):
):
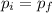 (1)
(1)
Being:
 (2)
(2)
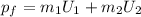 (3)
(3)
Where:
 is the mass of the first cart
is the mass of the first cart
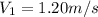 is the initial velocity of the first cart
is the initial velocity of the first cart
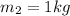 is the mass of the second cart
is the mass of the second cart
 is the initial velocity of the second cart
is the initial velocity of the second cart
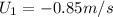 is the final velocity of the first cart
is the final velocity of the first cart
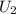 is the final velocity of the second cart
is the final velocity of the second cart
Substituting (2) and (3) in (1):
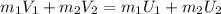 (4)
(4)
Isolating
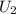 :
:
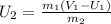 (5)
(5)
 (6)
(6)
Finally:
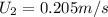 This is the speed of the second cart after the collision
This is the speed of the second cart after the collision