The inequality is 45 + 2x < 120.
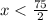
Solution:
Given a cost of video game = $45
Number of game controllers bought = 2
Each controller costs the same amount.
Total amount to spend = $120
Let x be taken the cost of game controller.
So, 45 + 2x < 120
Subtract 45 on both sides of the inequality.
⇒ 45 + 2x – 45 < 120 – 45
⇒ 45 – 45 + 2x < 120 – 45
⇒ 2x < 75
Divide by 2 on both sides of the inequality, we get
⇒
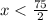
Hence, Kevin spend $
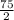 for each game controller.
for each game controller.