Question

You are given a (massless) piece of string that is L=1.45~\mathrm{m}L=1.45 m long, and tied to the end of it is an object of mass m=5.77~\mathrm{kg}.m=5.77 kg. Like any string, it will rip if there is too much tension in it, and for this string that maximal amount of tension is T_{\text{max}}=103~\mathrm{N}.T ​max ​​ =103 N. If you held this string at one end and twirled it in a circle above your head, so that the object moves in a plane parallel to the ground, what is the maximum speed that the object at the end of the string can have without breaking the string? (Hint: while the object moves in a plane parallel to the ground, the string cannot be parallel to the ground due to the presence of gravity.)

Answer 1

v = 4.25 m / s

Step-by-step explanation:

To solve this exercise we must use Newton's second law, let's set a reference system that is horizontal and vertical

Axis y

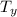`T_(y)` - W = 0

X axis

Tₓ = m a

The acceleration is centripetal

a = v² / r

Tₓ = m v² / r

v² = Tₓ r / m (1)

Let's use trigonometry to find the tension components,

sin θ = Tₓ / T

cos θ =
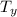`T_(y)`/ T

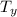`T_(y)` = T cos θ

Tₓ = T sin θ

Let's look angle for the maximum tension 103 N

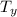`T_(y)` = T cos θ = W

θ = cos⁻¹ W / T

θ = cos⁻¹ (5.77 9.8 / 103)

θ = 56.7°

Now let's find the radius of the circle

sin 56.7 = r / L

r = L sin 56.7

We substitute in the speed equation (1)

v² = T sin 56.7 L sin 56.7 / m

v = √ T L sin² 56.7 / m

Let's calculate

v = √ (103 1.45 sin² 56.7 /5.77)

v = 4. 25 m / s