Question

If f(x) = (x3 − 4)7, then what is f '(x)?

Answer 1

To find the derivative f'(x) of the function
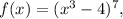`f(x) = (x^3 - 4)^7,`one must apply the chain rule. The chain rule gives us
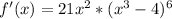`f'(x) = 21x^2 * (x^3 - 4)^6`as the solution.

Step-by-step explanation:

The question is asking to find the derivative of the function
`f(x) = (x^3 - 4)^7`. To solve this, we use the chain rule for differentiation, which states that the derivative of a composite function is the derivative of the outer function evaluated at the inner function multiplied by the derivative of the inner function. In this case, the outer function is
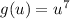`g(u) = u^7`and the inner function is
`h(x) = x^3 - 4.`

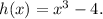
The derivative of
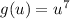`g(u) = u^7` with respect to u is
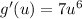`g'(u) = 7u^6`. The derivative of h
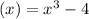`(x) = x^3 - 4`with respect to x is
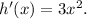`h'(x) = 3x^2.` Applying the chain rule, we get:

`f'(x) = g'(h(x)) * h'(x) = 7(h(x))^6 * h'(x) = 7(x^3 - 4)^6 * 3x^2`

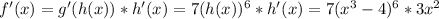
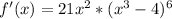`f'(x) = 21x^2 * (x^3 - 4)^6` is the derivative of the function f(x).