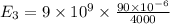Answer:
a)
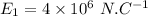
b)

Step-by-step explanation:
Given:
charge at the origin,
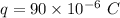
a)
Electric field at point
 :
:
The distance form the charge:
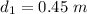
Now the electric field at the given point:

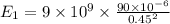
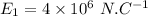
b)
Electric field at point
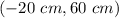 :
:
The distance form the charge:
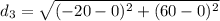
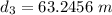
Now the electric field at the given point: