Answer:
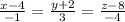
Explanation:
We are given that a point (4,-2,8)
Vector=<-1,3,-4>
We have to find the symmetric equation of line that passes through the point(4,-2,8) and parallel to given vector.
We know that the equation of line passing through the point (
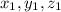 ) and parallel to vector <a,b,c> is given by
) and parallel to vector <a,b,c> is given by
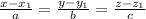
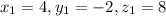 and <a,b,c>=<-1,3,-4>
and <a,b,c>=<-1,3,-4>
Using the formula
The symmetric equation of the line
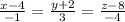
This is required symmetric equation of line.