Question

A small rock is thrown straight up from the edge of the roof of an 8.00 m tall building with an initial speed vo . The speed of the rock just before it strikes the ground is 24.0 m/s. What is the initial speed, vo , of the rock?

Answer 1

20.47 m/s

Step-by-step explanation:

t = Time taken

u = Initial velocity

v = Final velocity

s = Displacement

a = Acceleration

g = Acceleration due to gravity = 9.81 m/s² = a

`v^2-u^2=2as\\\Rightarrow s=(v^2-u^2)/(2a)\\\Rightarrow s=(24^2-0^2)/(2* 9.81)\\\Rightarrow s=29.3577\ m`

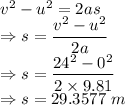
Total height of the fall is 29.3577 m

Height the ball reached above the building is
`29.3577-8=21.3577\ m`

`s=ut+(1)/(2)at^2\\\Rightarrow 21.3577=0t+(1)/(2)* 9.81* t^2\\\Rightarrow t=\sqrt{(21.3577* 2)/(9.81)}\\\Rightarrow t=2.0866\ s`

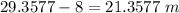
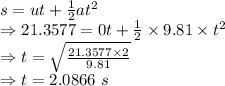
Time taken to reach the point from where the ball was thrown is 2.0866 s

This will also be the time it takes the ball to reach the maximum height

`s=ut+(1)/(2)at^2\\\Rightarrow u=(s-(1)/(2)at^2)/(t)\\\Rightarrow u=(21.3577-(1)/(2)* -9.81* 2.0866^2)/(2.0866)\\\Rightarrow u=20.47\ m/s`

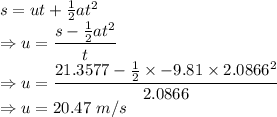
The initial velocity with which the rock was thrown was 20.47 m/s