The question is incomplete, here is the complete question:
A solution is prepared at 25°C that is initially 0.22 M in ammonia
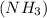 , a weak base with
, a weak base with
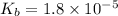 , and 0.073 M in ammonium chloride
, and 0.073 M in ammonium chloride
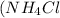 . Calculate the pH of the solution. Round your answer to 2 decimal places.
. Calculate the pH of the solution. Round your answer to 2 decimal places.
Answer: The pH of the solution is 9.74
Step-by-step explanation:
The chemical equation for the reaction of ammonia with hydrochloric acid follows:
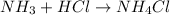
To calculate the pOH of basic buffer, we use the equation given by Henderson Hasselbalch:
![pOH=pK_b+\log(([salt])/([base]))](https://img.qammunity.org/2021/formulas/chemistry/college/whkb5aycrz2thv5lruk0dbi2nyi3z7t6qc.png)
![pOH=pK_b+\log(([NH_4Cl])/([NH_3]))](https://img.qammunity.org/2021/formulas/chemistry/college/hel6inuxbabb4kks1cyfm8u305acmcs7cw.png)
We are given:
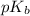 = negative logarithm of base dissociation constant of ammonia = 4.74
= negative logarithm of base dissociation constant of ammonia = 4.74
![[NH_4Cl]=0.073M](https://img.qammunity.org/2021/formulas/chemistry/college/o06fziewgve228hvu57ml8mki4j145kldk.png)
![[NH_3]=0.22M](https://img.qammunity.org/2021/formulas/chemistry/college/vffhfvp5oev2bibh3lcsf78o8ltr43jqj4.png)
pOH = ?
Putting values in above equation, we get:
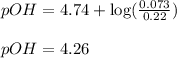
To calculate pH of the solution, we use the equation:
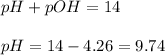
Hence, the pH of the solution is 9.74