Question

An escalator lifts people to the second floor of a building, 25 ft above the first floor. The escalator rises at a 30° angle. To the nearest foot, how far does a person travel from the bottom to the top of the escalator?

Answer 1

50 feet

Explanation:

Given:

Vertical height of lift by escalator (h) = 25 ft

Angle of inclination of escalator (x) = 30°

Now, the person traveling along the escalator is equal to the length of the escalator from bottom to top.

Now, a triangle can be constructed using the above scenario.

Consider a right angled triangle ABC with:

AB → Vertical height of escalator = 25 ft

AC → Length of escalator = ?

Now, using trigonometric ratio for sine, we can find the length AC.

This gives,

`\sin (x)=(AB)/(AC)\\\\\sin(30)=(25)/(AC)\\\\AC=(25)/(\sin(30))\\\\AC=(25)/(0.5)=50\ ft`

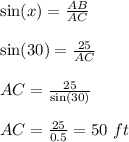
Therefore, the distance of travel of a person from bottom to top of the escalator is 50 feet.