Question

While standing at the edge of the roof of a building, a man throws a stone upward with an initial speed of 6.95 6.95 m/s. The stone subsequently falls to the ground, which is 14.3 14.3 m below the point where the stone leaves his hand. At what speed does the stone impact the ground? Ignore air resistance and use g = 9.81 m/s 2 g=9.81 m/s2 for the acceleration due to gravity.

Answer 1

18.1347 m/s

Step-by-step explanation:

t = Time taken

u = Initial velocity

v = Final velocity

s = Displacement

a = Acceleration

g = Acceleration due to gravity = 9.81 m/s² = a

`v^2-u^2=2as\\\Rightarrow s=(v^2-u^2)/(2a)\\\Rightarrow s=(0^2-6.95^2)/(2* -9.81)\\\Rightarrow s=2.4619\ m`

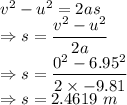
Total height the ball falls is 2.4619+14.3 = 16.7619 m

`v^2-u^2=2as\\\Rightarrow v=√(2as+u^2)\\\Rightarrow v=√(2* 9.81* 16.7619+0^2)\\\Rightarrow v=18.1347\ m/s`

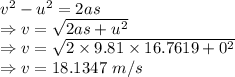
The speed at which the stone reaches the ground is 18.1347 m/s