Question

Talia has a daily budget of $94 for a car rental.write and solve an inequality to find the greatest distance talia can drive each day while staying within her budget

Answer 1

Explanation:

The Inequality representing the greatest distance Talia can drive each day is .

Answer 2

The Inequality representing the greatest distance Talia can drive each day is
`30+0.2x\leq 94`.

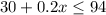Talia can drive maximum 320 miles each day while staying in her budget.

Explanation:

Image in the question was missing we have attached for the reference.

Given:

Daily budget for car rental of Talia = $94

Per day charge to rent a car =$30

Additional charge per mile = 0.20

We need to find how many miles can Talia drive each day.

Solution:

Let the number of miles Talia can drive each day be 'x'.

So we can say that;

Per day charge to rent a car plus Additional charge per mile multiplied by number of miles should be less than or equal to Daily budget for car rental of Talia.

framing in equation form we get;

`30+0.2x\leq 94`

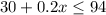
Hence the Inequality representing the greatest distance Talia can drive each day is
`30+0.2x\leq 94`.

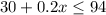On Solving the Inequality we get;

First we will subtract both side by 30 using Subtraction property of Inequality we get;

`30+0.2x-30\leq 94-30\\\\0.2x\leq 64`

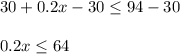
Now Dividing both side by 0.2 using Division property of Inequality we get;

`(0.2x)/(0.2)\leq (64)/(0.2)\\\\x\leq 320\ miles`

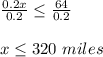
Hence Talia can drive maximum 320 miles each day while staying in her budget.