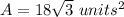Question

A disk with radius 3 units is inscribed in a regular hexagon. Find the approximate area of the inscribed disk using the regular hexagon

Answer 1

The approximate are of the inscribed disk using the regular hexagon is
`A=18√(3)\ units^2`

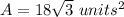
Explanation:

we know that

we can divide the regular hexagon into 6 identical equilateral triangles

see the attached figure to better understand the problem

The approximate area of the circle is approximately the area of the six equilateral triangles

Remember that

In an equilateral triangle the interior measurement of each angle is 60 degrees

We take one triangle OAB, with O as the centre of the hexagon or circle, and AB as one side of the regular hexagon

Let

M ----> the mid-point of AB

OM ----> the perpendicular bisector of AB

x ----> the measure of angle AOM

`m\angle AOM =30^o`

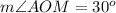
In the right triangle OAM

`tan(30^o)=((a/2))/(r)=(a)/(2r)\\\\tan(30^o)=(√(3))/(3)`

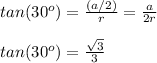
so

`(a)/(2r)=(√(3))/(3)`

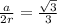
we have

`r=3\ units`

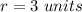
substitute

`(a)/(2(3))=(√(3))/(3)\\\\a=2√(3)\ units`

Find the area of six equilateral triangles

`A=6[(1)/(2)(r)(a)]`

simplify

`A=3(r)(a)`

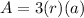
we have

`r=3\ units\\a=2√(3)\ units`

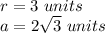
substitute

`A=3(3)(2√(3))\\A=18√(3)\ units^2`

Therefore

The approximate are of the inscribed disk using the regular hexagon is
`A=18√(3)\ units^2`