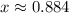Question

If ln(x)=−0.123, what does x equal? Express your answer numerically using three significant figures.

Answer 1

`\displaystyle x \approx 0.884`

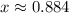
General Formulas and Concepts:

Symbols

• e (Euler's Number) ≈ 2.71

Pre-Algebra

• Equality Properties

Algebra I

• Exponential Rule [Rewrite]:
  `\displaystyle b^(-m) = (1)/(b^m)`

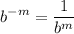
Algebra II

• Natural Logs ln and Euler's number e

Explanation:

Step 1: Define

Identify

`\displaystyle ln(x) = -0.123`

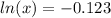
Step 2: Solve for x

1. [Equality Property] e both sides:
   `\displaystyle e^\bigg{ln(x)} = e^\bigg{-0.123}`
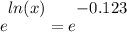
2. Simplify:
   `\displaystyle x = \frac{1}{e^\bigg{0.123}}`
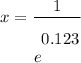
3. Evaluate:
   `\displaystyle x = 0.884264`
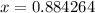
4. Round:
   `\displaystyle x \approx 0.884`