Step-by-step explanation:
It is known that formula for momentum per photon is as follows.
p =
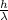
where,
 is the photon's wavelength.
is the photon's wavelength.
Putting the given values into the above formula as follows.
p =
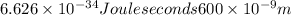
=

Therefore, the value of linear momentum is
 .
.
Now, energy per photon is calculated as follows.
E =
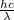
where, h = Planck's constant (
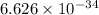 Joule seconds),
Joule seconds),
c = the velocity of light (
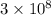 m/s).
m/s).
Hence, calculate the energy as follows.
E =
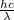
=
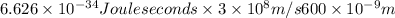
=
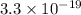 J
J
Hence, the value of energy per photon is
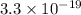 J.
J.
Now, we will calculate the energy per mole of photons as follows.
E =

where, E = the energy in a mole of photons,
N = Avogadro's number (
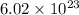 photons per mole),
photons per mole),
h = Planck's constant (
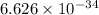 Joule seconds),
Joule seconds),
c = the velocity of light (
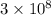 m/s)
m/s)
Putting these given values into the above formula and calculate the energy per mole of photons as follows.
E =

=
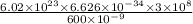
= 199 kJ/mol
Therefore, energy per mole of photons for radiation of wavelength for 600 nm (red) is 199 kJ/mol.