Question

string is fixed at two ends with distance 1.5 m. Its mass is 5 g and the tension in the string is 50 N and it vibrates on its third harmonic. a) What is the wavelength of waves of the string. b) What is the frequency of the waves. c) The vibrations

Answer 1

(a). The wavelength of waves of the string is 1 m.

(b). The frequency of the waves is 122.4 Hz.

(c). The wavelength of the sound emitted by the string is 2.8 m.

Step-by-step explanation:

Given that,

length of string = 1.5 m

Mass of the string = 5 g

Tension = 50 N

Suppose, The vibrations produce the sound with the same frequency. What is the wavelength of the sound emitted by the string

(a). We need to calculate the wavelength of waves of the string

Using formula of length

`L=(n\lambda)/(2)`

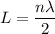
Here, n = 3

`L=(3\lambda)/(2)`

`\lambda=(2l)/(3)`

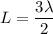
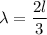
Put the value into the formula

`\lambda=(2*1.5)/(3)`

`\lambda=1\ m`

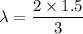
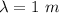
(b). We need to calculate the frequency

Using formula of frequency

`f=(v)/(\lambda)`

`f=\frac{\sqrt{(TL)/(m)}}{\lambda}`

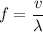
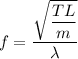
Put the value into the formula

`f=\frac{\sqrt{(50*1.5)/(5*10^(-3))}}{1}`

`f=122.4\ Hz`

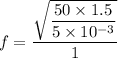
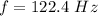
(c). We need to calculate the wavelength of the sound emitted by the string

Using formula of wavelength

`\lambda_(s)=(v)/(f)`

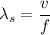
Put the value i to the formula

`\lambda_(s)=(343)/(122.4)`

`\lambda_(s)=2.8\ m`

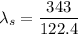
Hence, (a). The wavelength of waves of the string is 1 m.

(b). The frequency of the waves is 122.4 Hz.

(c). The wavelength of the sound emitted by the string is 2.8 m.