Question

A steam power plant operates on an ideal Rankine cycle with two stages of reheat and has a net power output of 120 MW. Steam enters all three stages of the turbine at 500°C. The maximum pressure in the cycle is 15 MPa, and the minimum pressure is 5 kPa. Steam is reheated at 5 MPa the first time and at 1 MPa the second time. Show the cycle on a T-s diagram with respect to saturation lines, and determine (a) the thermal efficiency of the cycle and (b) the mass flow rate of the steam.

Answer 1

a) 40.6%

b)72.19kg/s

Step-by-step explanation:

The Rankine cycle with two reheat stages has 9 stages in total.

The maximum pressure will be at the first inlet stage of the HP turbine which is stage 3. The minimum pressure will be the exit stage of the condenser because the condenser operates under vacuum pressure which is stage 1.

The following assumptions can be made:

1 - Each component in the cycle is analyzed as an open system operating at steady-state.

2 - All of the processes are internally reversible.

3 - The turbine and pump operate adiabatically and are internally reversible, so they are also isentropic.

4 - Condensate exits the condenser as saturated liquid.

5 - The effluent from the HP turbine is a saturated vapor.

6 - No shaft work crosses the system boundary of the boiler or condenser.

7 - Changes in kinetic and potential energies are negligible

a) The thermal efficiency of the cycle is defined as the work of the cycle divided by the total heat input to the system. The stages that have heat input is stages 2-3, 4-5, 6-7.

For stage 2:

s₁=s₂ assuming isentropic

`s_1=0.4762 @ P_1=15MPa`

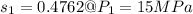
enthalpy will be a compressed liquid so after interpolation

`h_2=97.93+(0.4762-0.2932)((180.77-97.93)/(0.5666-0.2932))=153.38kJ/kg`

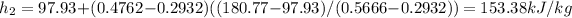
For stage 3:

Superheated steam @ T=500⁰C and P=15MPa

`h_3=3310.8kJ/kg`

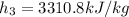
Stage 4:

superheated vapor

P=5MPa

s₃=s₄=6.3480 kJ/kg, we must use interpolation to find h₄

`h_4=2925.7-(6.348-6.2111)((3069.3-2925.7)/(6.4516-6.2111))=3007.44kJ/kg`

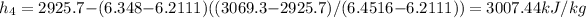
Stage 5:

Superheated steam @ T=500⁰C and P₄=P₅=5 MPa

`h_5=3434.7kJ/kg`

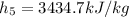
Stage 6:

Superheated steam at P₆= 1MPa

s₅=s₆

`s_6=6.9781`

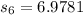
We find h₆ using interpolation from the steam tables:

`h_6=2943.1-(6.9781-6.9265)((3051.6-2943.1)/(7.1246-6.9265))=2970.67kJ/kg`

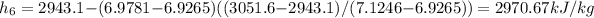
Stage 7:

P₇=P₆=1MPa

T=500⁰C superheated steam

`h_7=3479.1kJ/kg`

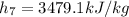
The heat into the cycle is:

`=(h_3-h_2)+(h_5-h_4)+(h_7-h_6)`

`=(3310.8-153.38)+(3434.7-3007.44)+(3479.1-2970.67)=4108.74kJ/kg`

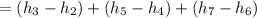
We can determine the work out by the condenser from stage 9 to stage 1:

Stage 1:

saturated liquid P=5kPa

`h_1=137.75kJ/kg`

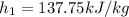
Stage 9:

We assume that its a saturated liquid with quality of 1 at 5kPa and

s₇=s₉ and after interpolation

`h_9=2568.53kj/kgK`

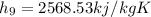
Qout = [/tex]2568.53-137.75=2430.79kJ/kg[/tex]

The thermal efficiency can be written in terms of qin and qout:

`n=1-(q_o/q_i)=1-2430.79/4093.11=0.4061`

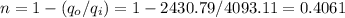
Efficiency of 40.61%

b)

The mass flow rate can be calculated from the Wnet:

`W_n=W_t-W_p`

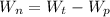
Work of the turbines minus the work of the pumps:

`W_n=m((h_3-h_4)+(h_5-h_6)+(h_7-h_9)-(h_1-h_2)`

`120000=m(1662.33)`

`m=72.19`

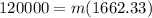
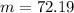
mass flow rate of steam is 72.19 kg/s