Answer:
Total number of lines formed by 6 points will be 15.
Explanation:
Number of lines determined by 2 points = 1
There is a condition that only 2 points lie on a line.
Total number of points on a plane = 6
We have to calculate the number of lines formed by the pairs of these 6 points.
It's a question of combinations.
Number of combinations formed =
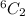
=
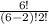
=
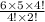
=
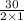
= 15
Therefore, total number of lines formed by 6 points will be 15.