Question

The heat of combustion of bituminous coal is 2.50  104 J/g. What quantity of the coal is required to produce the energy to convert 106.9 pounds of ice at 0.00°C to steam at 100.°C? specific heat (ice) = 2.10 J/g°C

Answer 1

This is an incomplete question, here is a complete question.

The heat of combustion of bituminous coal is 2.50 × 10² J/g. What quantity of the coal is required to produce the energy to convert 106.9 pounds of ice at 0.00 °C to steam at 100 °C?

Specific heat (ice) = 2.10 J/g°C

Specific heat (water) = 4.18 J/g°C

Heat of fusion = 333 J/g

Heat of vaporization = 2258 J/g

A) 5.84 kg

B) 0.646 kg

C) 0.811 kg

D) 4.38 kg

E) 1.46 kg

Answer : The correct option is, (A) 5.84 kg

Explanation :

The process involved in this problem are :

`(1):H_2O(s)(0^oC)\rightarrow H_2O(l)(0^oC)\\\\(2):H_2O(l)(0^oC)\rightarrow H_2O(l)(100^oC)\\\\(3):H_2O(l)(100^oC)\rightarrow H_2O(g)(100^oC)`

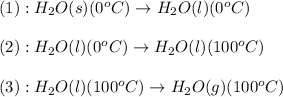
The expression used will be:

`Q=[m* \Delta H_(fusion)]+[m* c_(p,l)* (T_(final)-T_(initial))]+[m* \Delta H_(vap)]`

where,

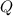`Q` = heat required for the reaction = ?

m = mass of ice = 106.9 lb = 48489.024 g (1 lb = 453.592 g)

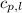`c_(p,l)` = specific heat of liquid water =
`4.18J/g^oC`

`\Delta H_(fusion)` = enthalpy change for fusion =
`333J/g`

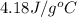
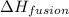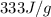
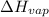`\Delta H_(vap)` = enthalpy change for vaporization =
`2258J/g`

Now put all the given values in the above expression, we get:

`Q=145903473.2J`

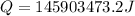
Now we have to calculate the quantity of the coal required.

`m=(Q)/(\Delta H)`

`m=(145903473.2J)/(2.50* 10^4J/g)`

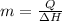
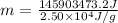
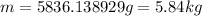`m=5836.138929g=5.84kg` (1 g = 0.001 kg)

Thus, the quantity of the coal required is, 5.84 kg