Question

Which of the following graphs represents a quadratic function f(x)=(x-3)^2+5

Answer 1

The graph in the attached figure

Explanation:

we have

`f(x)=(x-3)^2+5`

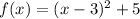
This is a vertical parabola open upward (the leading coefficient is positive)

The vertex represent a minimum

The vertex is the point (3,5)

The equation of the axis of symmetry of a vertical parabola is equal to the x-coordinate of the vertex

so

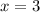`x=3` ---> axis of symmetry

Find the y-intercept (value of f(x) when the value of x is equal to zero)

For x=0

`f(x)=(0-3)^2+5=14`

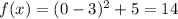
The y-intercept is the point (0,14)

Find the x-intercept (values of x when the value of f(x) is equal to zero)

For y=0

`0=(x-3)^2+5`

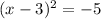`(x-3)^2=-5` ---> has no real solutions

The function has no x-intercepts (The roots of the quadratic equation are complex numbers)

therefore

The graph in the attached figure