Question

An old LP record that is originally rotating at 33.3 rad/s is given a uniform angular acceleration of 2.15 rad/s ².

Through what angle has the record turned when its angular speed reaches 72.0 rad/s?

Answer 1

`\theta = 947.7\ rad`

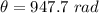
Step-by-step explanation:

given,

initial rotating speed = 33.3 rad/s

angular acceleration = 2.15 rad/s²

final angular speed = 72 rad/s

using equation of rotating wheel

`\omega_f = \omega_i + \alpha t`

`72 = 33.3+ 2.15 t`

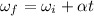
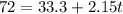
2.15 t = 38.7

t = 18 s

now, Again using equation of motion for the calculation of angle

`\theta = \omega_o t +(1)/(2)\alpha t^2`

`\theta = 33.3 * 18 +(1)/(2)* 2.15 * 18^2`

`\theta = 947.7\ rad`

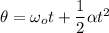
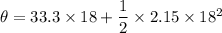
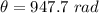
the angle record turned is equal to 947.7 radians.