Question

Solving a right triangle (round to the nearest tenth) ‼️‼️‼️

Answer 1

see explanation

Explanation:

The sum of the 3 angles in a triangle = 180°

Subtract the sum of the given angles from 180 for B

B = 180° - (90 + 47)° = 180° - 137° = 43°

-------------------------------------------------------------

tan47° =
`(opposite)/(adjacent)` =
`(a)/(14)`

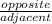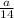
Multiply both sides by 14

14 × tan47° = a, thus

a ≈ 15.0 (to the nearest tenth )

---------------------------------------------------------------

cos47° =
`(adjacent)/(hypotenuse)` =
`(14)/(c)`

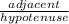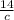
Multiply both sides by c

c × cos47° = 14 ( divide both sides by cos47° )

c =
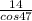`(14)/(cos47)` ≈ 20.5 ( to the nearest tenth )