Question

Given: A(g) + B(g) ⇋ C(g) + D(g)

At equilibrium a 2.00 liter container was found to contain 1.60 moles of C, 1.60 moles of D, 0.50 moles of A and 0.50 moles of B. Calculate Kc. enter a number to 2 decimal places

If 0.10 mole of B and 0.10 mole of C are added to this system, what is the value of Q? enter a number to 2 decimal places

What will the new equilibrium concentration of A be? enter a number to 3 decimal places

Answer 1

For 1: The value of
`K_c` is 10.24

For 2: The value of
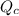`Q_c` is 9.07

For 3: The new equilibrium concentration of A is 0.220 M

Step-by-step explanation:

We are given:

Volume of the container = 2.00 L

Equilibrium moles of A = 0.50 moles

Equilibrium moles of B = 0.50 moles

Equilibrium moles of C = 1.60 moles

Equilibrium moles of D = 1.60 moles

We know that:

`\text{Molarity}=\frac{\text{Moles of solute}}{\text{Volume of solution}}`

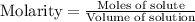
For the given chemical reaction:

`A(g)+B(g)\rightleftharpoons C(g)+D(g)`

• For 1:

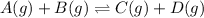
The expression of
`K_c` for above equation follows:

`K_c=([C][D])/([A][B])`

We are given:

`[A]_(eq)=(0.50)/(2.00)=0.25`

`[B]_(eq)=(0.50)/(2.00)=0.25`

`[C]_(eq)=(1.60)/(2.00)=0.8`

`[D]_(eq)=(1.60)/(2.00)=0.8`

Putting values in above equation, we get:

`K_c=(0.8* 0.8)/(0.25* 0.25)\\\\K_c=10.24`

Hence, the value of
`K_c` is 10.24

• For 2:

Added moles of B = 0.10 moles

Added moles of C = 0.10 moles

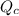`Q_c` is the quotient of activities of products and reactants at any stage other than equilibrium of a reaction.

`Q_c=([C][D])/([A][B])`

Now,

`[A]=(0.50)/(2.00)=0.25`

`[B]=(0.60)/(2.00)=0.3`

`[C]=(1.70)/(2.00)=0.85`

`[D]=(1.60)/(2.00)=0.8`

Putting values in above equation, we get:

`Q_c=(0.85* 0.8)/(0.25* 0.3)\\\\Q_c=9.07`

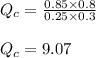
Hence, the value of
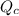`Q_c` is 9.07

• For 3:

Taking equilibrium constant as 10.24 for calculating the equilibrium concentration of A.

`K_c=10.24`

`[B]_(eq)=(0.60)/(2.00)=0.3`

`[C]_(eq)=(1.70)/(2.00)=0.85`

`[D]_(eq)=(1.60)/(2.00)=0.8`

Putting values in expression 1, we get:

`10.24=(0.85* 0.8)/([A]* 0.3)`

`[A]_(eq)=(0.85* 0.8)/(0.3* 10.24)=0.220`

Hence, the new equilibrium concentration of A is 0.220 M