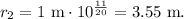Question

In general, if a sound has intensity of β dB at 1 m from the source, at what distance from the source would the decibel level decrease to 0 dB? Since the limit of hearing is 1 dB this would mean you could no longer hear it. Express the answer in terms of β. Possibly relevant information: The sound of normal breathing is not very loud, with an intensity of about 11 dB at a distance of 1 m away from the face of the breather. Again: EXPRESS THE ANSWER IN TERMS OF β!

Answer 1

Answer: The required distance is given by

`r_2=1\text{ m}\cdot 10^(\beta)/(20).`

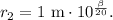
Explanation: The sound intensity in dB is given by the formula

`\beta \text{ dB}=10\log(I)/(I_0)`

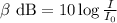
where
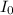`I_0` is the hearing threshold in absolute units and
`I` is the absolute intensity of the sound which depends on the distance. In general, for two distances
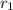`r_1` and
`r_2` we have that

`(I(r_1))/(I(r_2))=(r_2^2)/(r_1^2)=\left((r_2)/(r_1)\right)^2.`

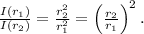
Now let us take
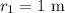`r_1=1\text{ m}` and let
`r_2` be the required distance. We have

`\beta \text{ dB}=10\log(I(r_1))/(I_0),\quad 0\text{ db}=10\log(I(r_2))/(I_0).`

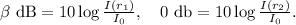
Exponentiating these equations we obtain

`10^{(\beta)/(10)}=(I(r_1))/(I_0),\quad 10^0=1=(I(r_2))/(I_0).`

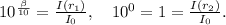
Dividing them

`(I(r_1))/(I(r_2))=10^(\beta)/(10).`

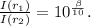
Using the previously stated identity

`(r_2^2)/(r_1^2)=10^(\beta)/(10)\Rightarrow r_2=r_110^(\beta)/(20)=1\text{ m}\cdot 10^(\beta)/(10)`

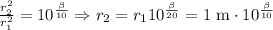
Now if we use the given example where
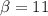`\beta=11` we have

`r_2=1\text{ m}\cdot 10^{(11)/(20)}=3.55\text{ m}.`