Question

Find the maximum value or minimum value for the function f(x) = 0.15(x + 1)² - 3.

0.15
3
1
-3

Answer 1

The minimum value for
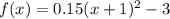`f(x) = 0.15(x + 1)^2 - 3` is
`-3`.

Explanation:

Given function is
`f(x) = 0.15(x + 1)^2 - 3`

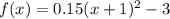
We need to find the maximum value or the minimum value for the function.

Now, differentiate
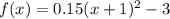`f(x) = 0.15(x + 1)^2 - 3` w.r.t
`x`.

`f'(x) =(d)/(dx)(0.15(x + 1)^2 - 3)\\f'(x)=(d)/(dx)(0.15(x+1)^2-(d)/(dx)(3)\\`

`f'(x)=2* 0.15(x+1)(d)/(dx)(x+1)-0\\f'(x)=0.3(x+1)(1)\\f'(x)=0.3(x+1)`

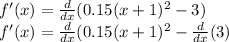
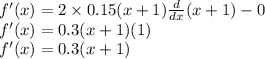
Now, we will equate
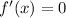`f'(x)=0` to find critical point.

`0.3(x+1)=0\\x=-1`

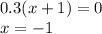
Plug this critical point in to the function
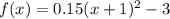`f(x) = 0.15(x + 1)^2 - 3` we get,

`f(-1) = 0.15(-1 + 1)^2 - 3\\f(-1)=-3`

Also,
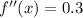`f''(x)=0.3` which is positive, We have minimum value.

So, the minimum value for
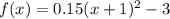`f(x) = 0.15(x + 1)^2 - 3` is
`-3`.