Answer: The specific heat of beryllium is 1.83 J/g°C
Step-by-step explanation:
To calculate the mass of water, we use the equation:

Density of water = 1.00 g/mL
Volume of water = 59.1 mL
Putting values in above equation, we get:
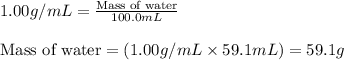
When berrylium is dipped in water, the amount of heat absorbed by metal will be equal to the amount of heat released by water.
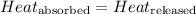
The equation used to calculate heat released or absorbed follows:
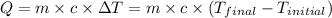
![m_1* c_1* (T_(final)-T_1)=-[m_2* c_2* (T_(final)-T_2)]](https://img.qammunity.org/2021/formulas/chemistry/college/8mq914tycmkoswztk5p5my04anxw73dmzg.png) ......(1)
......(1)
where,
q = heat absorbed or released
 = mass of water = 24.7 g
= mass of water = 24.7 g
 = mass of beryllium= 59.1 g
= mass of beryllium= 59.1 g
 = final temperature = 32.0°C
= final temperature = 32.0°C
 = initial temperature of water = 20.2°C
= initial temperature of water = 20.2°C
 = initial temperature of beryllium =96.7°C
= initial temperature of beryllium =96.7°C
 = specific heat of water= 4.186 J/g°C
= specific heat of water= 4.186 J/g°C
 = specific heat of beryllium = ?
= specific heat of beryllium = ?
Putting values in equation 1, we get:
![59.1* 4.186* (32.0-20.2)=-[24.7* c_2* (32.0-96.7)]](https://img.qammunity.org/2021/formulas/chemistry/college/2sdwelflpsca0p0nbqylz89nh70h262y5o.png)
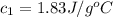
Hence, the specific heat of beryllium is 1.83 J/g°C