Question

At a distance r1 from a point charge, the magnitude of the electric field created by the charge is 344 N/C. At a distance r2 from the charge, the field has a magnitude of 171 N/C. Find the ratio r2/r1.

Answer 1

The ratio of distances r2/r1 from a point charge where the electric fields are 171 N/C and 344 N/C is found using the inverse square law of electric fields and is approximately sqrt(2) or 1.414.

Step-by-step explanation:

The question involves finding the ratio of two distances r2 and r1 from a point charge where the electric field magnitudes are 171 N/C and 344 N/C, respectively. According to the inverse square law, the magnitude of the electric field (E) created by a point charge is proportional to the inverse square of the distance (r) from the charge. The electric field is given by E = kQ/r^2, where k is Coulomb's constant and Q is the charge. The ratio of the squares of the distances will be inversely proportional to the ratio of the electric fields. Therefore, if E1 is 344 N/C at r1 and E2 is 171 N/C at r2, we get the ratio E1/E2 = r2^2/r1^2 = 344/171. Simplifying this, we find that r2/r1 = sqrt(344/171), which is approximately sqrt(2) or 1.414.

Answer 2

To solve this problem we will apply the concepts related to the electric field. This definition is based on the proposal by Coulomb and will be described in its two states. Under this relationship we will obtain the radius of the distances depending on the elective field.

The electric field strength of the charge from a distance
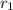`r_1` is,

`E_1 = k (Q)/(r_1^2)`

The electric field strength of the charge from a distance
`r_2` is,

`E_2 = k (Q)/(r_2^2)`

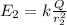
The relation between the electric field strengths
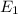`E_1` and
`E_2` is

`(E_1)/(E_2)` and can be written as

`(E_1)/(E_2) = (k (Q)/(r_1^2))/(k (Q)/(r_2^2))`

`(E_1)/(E_2) = (r_2^2)/(r_1^2)`

`(r_2)/(r_1) = \sqrt{(E_1)/(E_2)}`

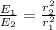
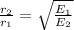
Replacing we have,

`(r_2)/(r_1) = \sqrt{(344N/C)/(177N/C)}`

`(r_2)/(r_1) = 1.39`

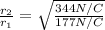
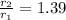
The ratio of the distances
`r_2` and
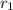`r_1` is 1.39