Question

Find a positive number for which the sum of it and its reciprocal is the smallest​ (least) possible.

Answer 1

1 is the positive number for which the sum of it and its reciprocal is the smallest.

Explanation:

Let x be the positive number.

Then, the sum of number and its reciprocal is given by:

`V(x) = x + (1)/(x)`

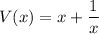
First, we differentiate V(x) with respect to x, to get,

`(d(V(x)))/(dx) = (d(x+(1)/(x)))/(dx) = 1-(1)/(x^2)`

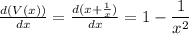
Equating the first derivative to zero, we get,

`(d(V(x)))/(dx) = 0\\\\1-(1)/(x^2)= 0`

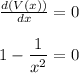
Solving, we get,

`x^2 = 1\\x= \pm 1`

Since x is a positive number x = 1.

Again differentiation V(x), with respect to x, we get,

`(d^2(V(x)))/(dx^2) = (2)/(x^3)`

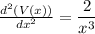
At x = 1

`(d^2(V(x)))/(dx^2) > 0`

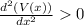
Thus, by double derivative test minima occurs for V(x) at x = 1.

Thus, smallest possible sum of a number and its reciprocal is

`V(1) = 1 + (1)/(1) = 2`

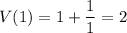
Thus, 1 is the positive number for which the sum of it and its reciprocal is the smallest.